Uncertainty in measurement may often occur in different experimental and theoretical calculations. Numbers are used conveniently to focus on the possible results in experiments with some amount of uncertainty. The impact of uncertainty in different types of measurements has been defined as a parameter that is associated with the outcomes of the specific measurement in chemistry that traits the distribution of values.
Chemist has observed that at different times systematic errors can be related to the effects of scientific factors. Additionally, it has been observed that there are two different kinds of uncertainties in measurement regarding engineering variables, are random uncertainty and systematic uncertainty. All measuring devices have some uncertainty degree.
Figure 1: Probability density of measurement
D Wells, Standard Normal Distribution, CC BY-SA 4.0
The different procedures of measurement in chemistry have defined the attributes or properties of substances.
The followings are the additional part of the specific notation in chemistry to comprehend uncertainty in measurement −
Molecules and atoms have excessive low masses, however; these are still available in large numbers.
Chemists even negotiate with figures for the mass of hydrogen atoms that can be as little as 0.00000000000000000000000166g. Even chemistry has focused on further experiment regarding the process of handling the numbers.
In order to manage the small or large numbers, the notation: x 10y is used where the representation is made based on the x times ten raised to the power of y. Additionally, in the above furnished expression, y is an exponent that includes either negative or positive values and x is a digit that value can vary from 1.000 to 9.999.
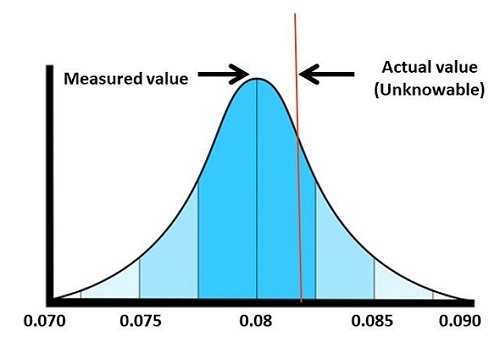
Figure 2: Measurement uncertainty
The expression of the scientific notation of 823.912 also expressed as the scientific notation 8.23912 × 102. The expression defines that the decimal is shifted to the left side by two positions and if it is shifted three positions to the left, then the power of 10 should be 3. Moreover, with the same desire, 0.00075 can be expressed as 7.5 × 10-4, in this expresses the decimal is shifted to the four positions on the right side. Additionally, −4 referred to the exponent in the scientific notation.
Different equations in chemistry often followed different mathematical equations for the development of different exponents. Different operations in chemistry have the urge to place the numbers in different ways that have identical exponents. Therefore, 5.43 × 104 and 3.45 × 103 are defined by the power are equal but the coefficients are added are different and the subtraction is done by following the method of simple addition-substraction rule in the uncertainty measurement. The method is as follows −
$$\mathrm{5.43 × 10^4 – 0.345 × 10^4 = [5.43 – (0.345)]× 10^4 = (5.43 – 3.45) × 10^4 = 1.98 × 10^4}$$
The above expression is mentioned in the subtraction case to get a better understanding of the uncertainty in measurement.
The outcome from any uncertainty formula can be measured in in a percentage notation or simple ratio. Thus, it can be estimated as the following formula −
$$\mathrm{Percent\:uncertainty = Uncertainty / Actual\:value\:\times\:100}$$
For example, a scale can be estimated as potentially wrong value as 6 cm due to additional marginal issues. The outcome of the measurement can be the same as 10 cm where the factors can be calculated as percentage error.
Experimental errors may be separated into two different classes, such as, systematic errors and random errors. Based on the process and quantity, the calculation procedure is referred to as accuracy as a qualitative notion that express the agreement between a measured value and a true value of a measured. Additionally, bias is the primary phrase used to explain the magnitude of any systematic error.
The following table represents the primary distinctions with regard to systematic and random errors −
| Systematic Errors | Random Errors |
|---|---|
| Systematic error can theoretically be destroyed from the outcome of an appropriate correction. | Random errors originate from variations that are unpredictable with the influence of the procedure of measurement. |
| Systematic errors are derived from non-statistical data | Random errors can be statistically analysed |
| The adaption of the GUM process helps in analysing the systematic error. | Internal quality control procedures are used to evaluate the random errors. |
Table 1: Different between systematic and random Errors
The selection of rules for the calculation of functional properties refers to the uncertainty. Different scientific applications in chemistry want to use different types of calculation for the development of the trigonometric functions. However, the process of uncertainty is associated with the system of measurement that is propagation with the definition of functional relationship.
Measurement is the phase that denotes to quantify measuring values. The application of uncertainty measurement in chemistry follows the specification where it is required. There are often some circumstances under which a measuring activity can be executed. ISO 15189 has defined the specific meaning and requirement for the uncertainty of measurement.
Q1. What is uncertainty measurement?
Ans. Measurement uncertainty refers to a parameter that is often employed in the processing of data for the description of both the distribution of the outcome and its calculated contrast from the accurate value. Additionally, error and uncertainty are the two different classes based on the possible outcome of the measured value.
Q2. What is the key difference between precision and accuracy?
Ans. Accuracy is defined as the degree of closeness to true value while precision refers to the degree to which a mechanism will repeat with identical value while performing the experiment.
Physics
Biology
Chemistry
Mathematics
Economics
Psychology
Environmental Science
Social Studies
Fashion Studies
English