

Average Speed and Average Velocity are two important concepts of kinematics. The ratio between distance cover by an object and time taken to cover is called Speed.
Velocity is the ratio of displacement of an object and time taken. Speed is a scalar quantity. Velocity is a vector quantity. Magnitude and direction should be known to define velocity as a vector quantity. The difference between speed and velocity is that velocity is the speed with direction. For example, a bike travels at 50km\h shows its speed. A bike travels at 50km\h in the east represents its velocity.
$$\mathrm{Speed= s=\frac{Distance}{time}}$$
$$\mathrm{Velocity= v=\frac{Displacement}{time}}$$
Ratio between total distance travelled and total time taken is called Average Speed. Distance is defined as a change in the position of an object. But in a specific direction, a change of position is called Displacement. In an equal period of time, an object covers the unequal distances then its velocity is said to be variable. But in an equal period of time a body covers an equal distance then its velocity is said to be uniform.
The ratio between the change in displacement and the time intervals when displacement happens is called Average Velocity. The sign of the displacement decides whether the average velocity is positive or negative. A meter per second is the SI unit of Average velocity. It is represented as ms-1. It is also a vector quantity. Change in displacement is represented as Δx and time interval is represented as Δt.
$$\mathrm{Average\:velocity= \overrightarrow{v}=\frac{\Delta x}{\Delta t}m/s}$$
The formula for finding the average velocity,
Average velocity $\mathrm{\overrightarrow{v}=\frac{\Delta x}{\Delta t}m/s}$
Changes in displacement
$$\mathrm{\Delta x=x_f-x_i}$$
$$\mathrm{x_i -initial\:displacement}$$
$$\mathrm{x_f-final\:displacement}$$
Time interval
$$\mathrm{\Delta t=t_f-t_i}$$
$$\mathrm{t_i-ending\:time}$$
$$\mathrm{t_f-starting\:time}$$
Therefor
$$\mathrm{\overrightarrow{v}=\frac{\Delta x}{\Delta t}=\frac{x_f-x_i}{t_f-t_i}}$$
$$\mathrm{\Delta x-change\:in\:displacement}$$
$$\mathrm{\Delta t-total\:time}$$
$$\mathrm{\overrightarrow{v}-Average\: velocity}$$
Average velocity Examples
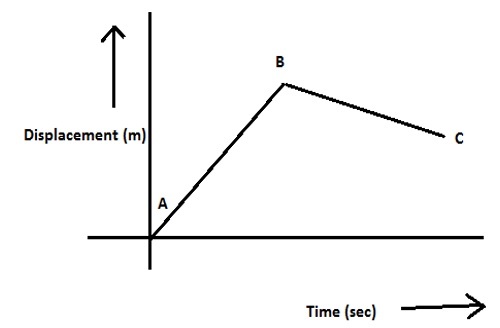
In the image, an object moving from A to B is the initial displacement then it moves from B to C is the final displacement. The X-axis and the Y-axis represents the displacement and time respectively. Starting time is zero. Let the initial displacement is 45.4m and the final displacement is 36m. The total time taken by the object is 4 sec.
When starting time is zero, the average velocity will be
$$\mathrm{\overrightarrow{v}=\frac{\Delta x}{t}}$$
$$\mathrm{\Delta x=x_f-x_i}$$
Given data:
$$\mathrm{x_f=36\:m}$$
$$\mathrm{x_i=45.4\:m}$$
$$\mathrm{t=4\:sec}$$
$$\mathrm{\overrightarrow{v}=\frac{36-45.4}{4}=-\frac{9.4}{4}=-2.35\:ms^{−1}}$$
Average velocity is -2.35m/s. We already know that the Sign of the displacement decides whether the average velocity is positive or negative. Here the sign of the displacement is negative so the average speed value is also negative.
| Average Speed | Average Velocity |
|---|---|
| Ratio between total distance travelled and total time taken is called Average speed. | The ratio between the change in displacement and the time intervals when displacement happens is called Average Velocity. |
| There is no particular direction for average speed. So, it is a scalar quantity. | Average Velocity always depends on the direction. So, it is a vector quantity. |
| Average Speed is permanently positive. | Average Velocity is either positive or negative. Because average velocity depends on direction and displacement. |
| Average Speed $\mathrm{S=d/t\:ms^{−1}}$ | Average Velocity $\mathrm{\overrightarrow{v}=\frac{\Delta x}{\Delta t}m/s}$ |
| Example: A bike traveling at a speed of 40km/h. | A bike traveling at a velocity of 40 km/h south. |
Table-1: The difference between Average Speed and Average Velocity
The average velocity is zero when an object tries to return to the starting stage after displacement or an object at a rest point. In other words, Displacement of an object is zero then the average velocity becomes zero. For example, a car travels from point A to point B and returns to point A. In this case speed of a car can be calculated but velocity cannot be calculated because displacement is zero. But a car travel from point A to point B and settles at point B. In this case, displacement is calculated. So, there is no chance for the average velocity to become zero in that situation.
Speed is defined as the change ratio of position of a particle. The change ratio of the position of the particle taking place in a specific direction is called velocity. The ratio between total distance travelled and total time taken is called Average Speed. The ratio between the change in displacement and the time intervals when displacement happens is called Average Velocity. Angular velocity is also defined as the difference of change in angular displacement. The ratio of change in angular velocity with time is defined as Angular acceleration. Displacement of an object is zero then average velocity becomes zero. SI unit for Average Speed and Average Velocity is meter per second $\mathrm{(ms^{−1})}$.
Q1. A railway train 200m long, passes over a bridge 800m long. Find the time it takes to cross the bridge when it is moving with a uniform velocity of 36km/h.
Ans. Given
$$\mathrm{Velocity=36\:km/h}$$
$$\mathrm{v=\frac{36×1000}{3600}=10\:m/s}$$
Total distance to be covered $\mathrm{=d=200+800=1000\:m}$
$$\mathrm{Velocity\:v=\frac{d}{t}}$$
$$\mathrm{t=\frac{d}{v}\:sec}$$
Time required to cross the bridge $\mathrm{=\frac{1000}{10}=100\:sec}$
Q2. Define Scalar and Vector Quantity.
Ans. Physical quantity with magnitude and without direction is called Scalar quantity. Speed, time, volume, temperature, distance, and density are examples of a scalar quantity. A quantity that has both size and direction is called a vector quantity. Velocity, angular velocity, force, electric field, linear momentum, and polarisation are examples of a vector quantity. The size of a vector is called the modulus of the vector.
Q3. What is Angular Velocity?
Ans. The angular velocity of the body is defined as the angle described by it per second about the axis of rotation. It is a vector quantity. Angular velocity is the angle made by the body in time t.
Angular Velocity = $\mathrm{ω=\frac{\theta}{t}\:rad/sec}$
Here
θ= angle made by the body
t=time
Q4. What is the relation between acceleration and velocity?
Ans. The ratio of change in velocity of the body and time is called Acceleration. It is a vector quantity. Because it has both size and direction.
$$\mathrm{Acceleration,\:a=\frac{v_f-v_i}{t}}$$
$\mathrm{v_f}$ =final velocity
$\mathrm{v_i}$ =initial velocity
t=time (sec)
Q5. An object travels half of its period of traveling with a speed of a m/s and the other half with a speed of b m/s. Calculate the average speed of the body during the whole travel of the object.
Ans. 2D is the total distance covered by the body. $\mathrm{t_1}$ and $\mathrm{t_2}4 is the time of the body to cover in the first half and second of respectively. S is the average speed.
Normally,
$$\mathrm{Average\:speed \:S=\frac{d}{t}}$$
a is the speed of the first half. b is the speed of the other half. So from the formula of speed, we should know that,
$$\mathrm{t=\frac{2D}{a}}$$
$$\mathrm{t_1=\frac{D}{a};\:t_2=\frac{D}{b}}$$
$$\mathrm{Total\:time\:t=t_1+t_2}$$
$$\mathrm{t=\frac{D}{a}+\frac{D}{b}=D(\frac{1}{a}+\frac{1}{b})}$$
$\mathrm{\therefore}$ Equating the both equation of t
$$\mathrm{\frac{2D}{s}=D(\frac{1}{a}+\frac{1}{b})}$$
$$\mathrm{Average\:Speed=S=\frac{2ab}{a+b}m/s}$$